Home
Saving
Investing
Business
Math
Macroeconomics
Blog
Install CalcoPolis as app
Inflation calculator
How will inflation decrease the value of your assets?
Compound Interest Calculator
With compound interest, saving is easier than you think.
What is compound interest?
Compound interest is a straightforward yet compelling concept. It represents the cumulative growth of the initial savings with interests accumulated over time.
The idea of "interests on the interests" is the basis for most popular financial products, like saving accounts, deposits, etc. Thanks to this a small capital can grow over time to a huge value.
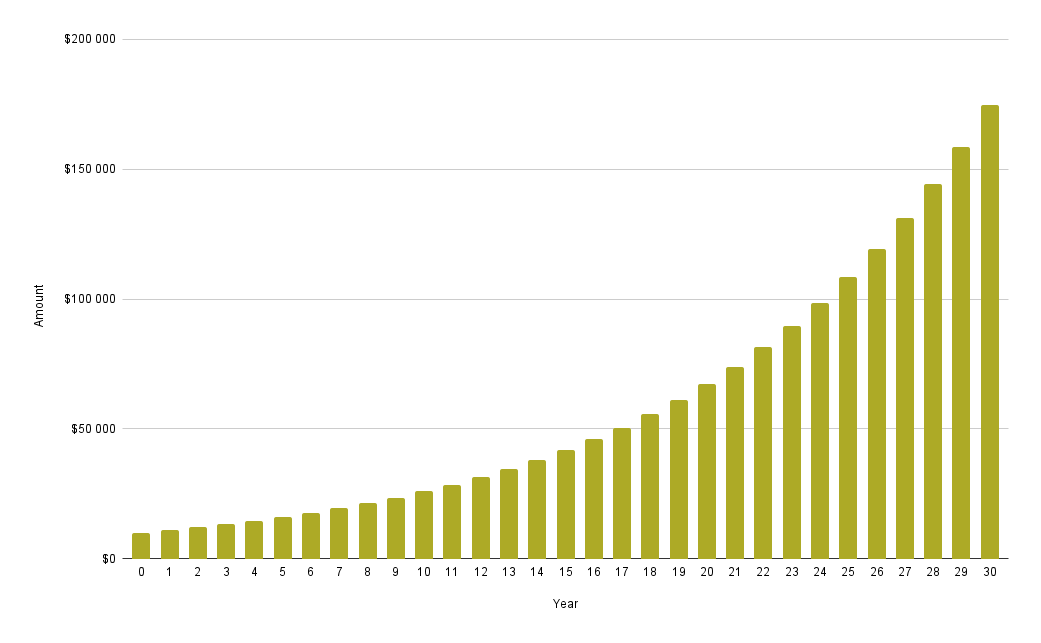
Look at this sample compound interest chart representing cumulative growth of $1000 over 30 years with a 10% interest rate.
How to calculate compound interest?
The value of compound interest can be calculated using the following standard formula
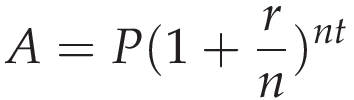
Where:
- A = the future value of the initial amount
- P = the initial amount.
- r = the annual interest rate as a decimal number
- n = the number of times that interest is compounded per year
- t = the number of years the money is invested. (If you wish for a shorter period, you can substitute 1/12 for one month)
As a result of this formula, you will receive the final value of your investment with all accumulated interests.
If you want to calculate the accumulated interest's exact value, you can simply subtract the initial amount from this result.
According to the above formula, the future value depends on the following:
- time of investment
- interest rate
- total number of compounding periods
- initial amount
Of course, a manual calculation using this formula is a very daunting and time-consuming task. That's why we created this free online calculator.
Using this interest calculator
If you wish to find the future value of your deposit or any other investment, simply fill in the form above and click the button Calculate.
Initial Investment
The starting value of your deposit. The results are presented in US dollars, but in fact, it does not have any impact on the calculated values. So you can use it for Euro, Yen, Swiss Franc, and any other currency.
Interest Rate
The annual value of the interest rate of your investment.
Period
How long do you plan to keep your deposit?
Compounding
How often are the accumulated interests added to the base of the future interest accrual? The more frequent the compounding the bigger the growth of the future value.
Tax rate
The value of income tax in your country.
Our tool makes it very easy to calculate compound interest and adds many useful features.
- Calculating the income tax
- Calculation of the impact of inflation
- Detailed compounding table
- Colorful charts and detailed tables
Compound interest example
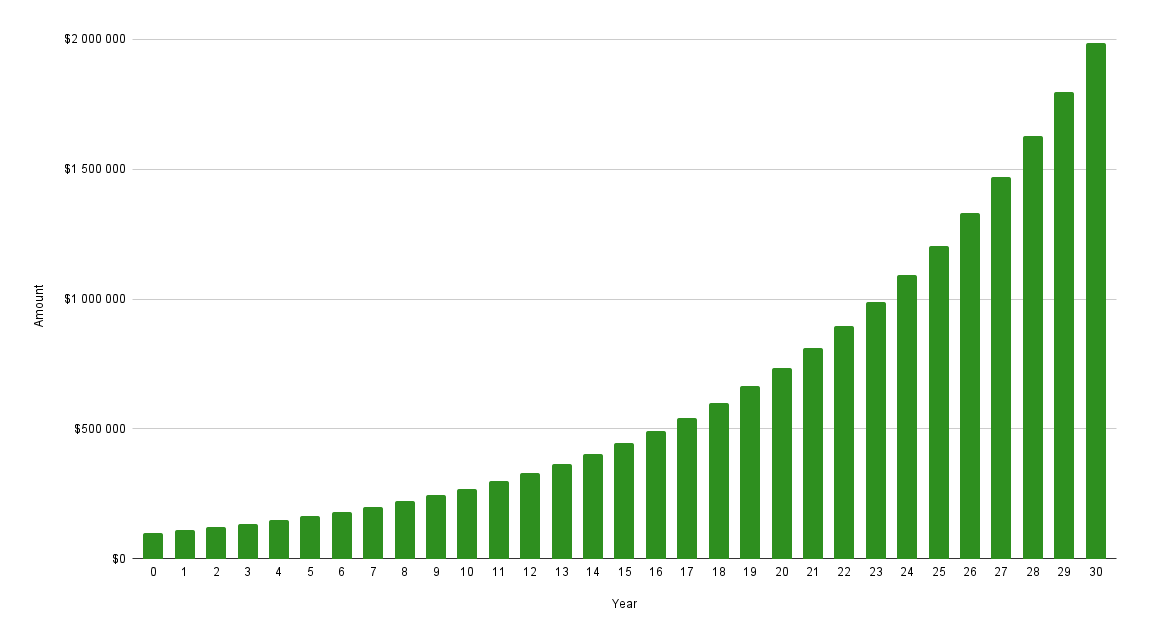
To better illustrate the power of compounding interest, we prepared the simulation of the growth of an initial amount of $100000 over 30 years at 10% per year.
In order to demonstrate a more real-life scenario, we use monthly compounding of interest because it is standard in most banks.
| Year | Initial amount | Interests | Final amount |
| 1 | $100 000 | $10 471 | $110 471 |
| 2 | $110 471 | $11 568 | $122 039 |
| 3 | $122 039 | $12 779 | $134 818 |
| 4 | $134 818 | $14 117 | $148 935 |
| 5 | $148 935 | $15 595 | $164 531 |
| 6 | $164 531 | $17 229 | $181 759 |
| 7 | $181 759 | $19 033 | $200 792 |
| 8 | $200 792 | $21 026 | $221 818 |
| 9 | $221 818 | $23 227 | $245 045 |
| 10 | $245 045 | $25 659 | $270 704 |
| 11 | $270 704 | $28 346 | $299 050 |
| 12 | $299 050 | $31 314 | $330 365 |
| 13 | $330 365 | $34 594 | $364 958 |
| 14 | $364 958 | $38 216 | $403 174 |
| 15 | $403 174 | $42 218 | $445 392 |
| 16 | $445 392 | $46 638 | $492 030 |
| 17 | $492 030 | $51 522 | $543 552 |
| 18 | $543 552 | $56 917 | $600 469 |
| 19 | $600 469 | $62 877 | $663 346 |
| 20 | $663 346 | $69 461 | $732 807 |
| 21 | $732 807 | $76 735 | $809 542 |
| 22 | $809 542 | $84 770 | $894 311 |
| 23 | $894 311 | $93 646 | $987 958 |
| 24 | $987 958 | $103 452 | $1 091 410 |
| 25 | $1 091 410 | $114 285 | $1 205 695 |
| 26 | $1 205 695 | $126 252 | $1 331 946 |
| 27 | $1 331 946 | $139 472 | $1 471 419 |
| 28 | $1 471 419 | $154 077 | $1 625 495 |
| 29 | $1 625 495 | $170 211 | $1 795 706 |
| 30 | $1 795 706 | $188 034 | $1 983 740 |
Key takeaways from this chart:
- With a 10% interest rate, the value of the investment doubles every seven years.
- Over time compound interest grows at an ever-increasing rate. Each subsequent period gives you a higher return.
- It takes 7 years to earn the first 100k of interest, but earning the final 100k takes only seven months.
Real-life applications of compound interest
Below you will find examples of investments using this idea. Compound interest is the root of every financial asset.
Certificates of deposit
This is the most basic example. Your deposit will grow in value according to your interest rate.
Saving accounts
Saving accounts are also very intuitive examples; this kind of financial instrument allows you to add extra deposits whenever you want. Such additional payments add some complexity to the calculations, but you can evaluate them using our savings calculator.
Stocks
Stocks are another real-life example of the power of compound interest. Of course, when buying stock, there is no fixed interest rate, but since most companies grow over time, their stock grows in value accordingly.
Index and mutual funds
Managed funds similarly grow in value as well. Interest rates may vary, and as in the case of stock, there might be periods of negative returns, but over a long period, the idea still applies.
Complex Portfolios
When forecasting possible return rates of portfolios composed of different classes of assets, you may use a compound interest formula with an assumed average annual return rate.
Inflation
This is an example of compound interest with a negative interest rate. For more details visit our inflation calculator.
FAQ
What is a compounding frequency?
While your savings can generate interest daily, most financial instruments add earned interest to the account balance at fixed periods — for example, every month. This is called compounding frequency. The most common cases are:
- daily compounding
- monthly compounding
- quarterly compounding
- yearly compounding
The more often your interests are compounded, the more profit you gain because each penny will start working for you sooner.
What is the effective annual interest rate?
The effective annual interest rate, including compounding frequency, represents the actual interest rate. If the accrual of interest occurs more often than once per year, the actual interest rate is higher than the nominal interest rate.
Similar tools
On Calcopolis, you can find many more useful tools for savvy people who actively work to increase their net worth.
For example, you may check if you have accumulated enough money to live off your capital (visit the living off savings calculator).
If you wonder how long it will take to save a specific amount of money at your savings rate, to do so, visit our savings goal calculator.
Authors
Created by Lucas Krysiak on 2022-02-15 13:54:08 | Last review by Mike Kozminsky on 2022-08-24 15:05:28
